Data Update 5: Relative Risk and Hurdle Rates

In my last four posts, I focused on the macro variables that we draw on, in both corporate finance and valuation, to estimate required returns or hurdle rates. In data post 3, I looked at how the prices of risk in both the bond market (default spreads) and the equity market (equity risk premiums) dropped in 2019, in the US. In data post 4, I extended the discussion to cover country and currency risk. In this one, I will bring in the micro variables that cause differences in risk across firms, and how to convert them into risk measure.

Relative Risk Measures

To get from the macro risk measures to company-level hurdle rates, you need to make judgments on relative risk. Put simply, if you buy into the proposition, like I do, that some companies/investments are riskier than others, you need a measure of relative risk that captures this variation.  It is in this context that I think of betas, a loaded concept that carries with it the baggage of modern portfolio theory and efficient markets. If you add to this the standard approach to estimating betas, built on looking at past prices and running regressions against market indices, you have the makings of a perfect storm, designed to drive value investors to apoplexy. I have no desire to re-litigate these arguments, partly because those for and opposed to betas are set in their ways, but let me suggest some compromise propositions.

Relative Risk Proposition 1: You do not need to believe in betas to do financial analysis and valuation. 

While there are many who seem to tie discounted cash flow valuations to the use of beta or betas, there is nothing inherently in  a DCF that requires that you make this leap:

While the discount rate in a DCF is a risk-adjusted number, the approach is agnostic about how you measure risk and adjust discount rates for that risk.

Relative Risk Proposition 2: If you don't like to or want to measure relative risk with betas, you can come up with alternate measures that better reflect your view of how risk should be measured. 

While I do use beta as my proxy for risk, I do so with open eyes, recognizing its many limitations as a risk measure, and I have been always willing to consider competing risk measures. In fact, I have presented alternate measures of risk, drawing on the two building blocks of betas that draw the most pushback. The first is the assumption that marginal investors are diversified, and that the only risk that needs to be measured is the risk that cannot be diversified away. The second is its use of prices (stock and market) to estimate risk, seemingly contradicting intrinsic value's basic precept that market prices are not trustworthy. Since a picture is worth a thousand words, here a few alternative risk measures to consider, if you don't trust betas;

Put simply, if your primary problem with betas is the assumption that marginal investors are diversified, there are total risk measures that are built around measuring the total risk in a company or investment, by looking at either the standard deviation or adding premiums (small cap, company-specific risk) to the traditional risk and return model. If your concern is that past prices are being used to estimate betas, you can switch to using accounting earnings and computing risk measures either from the perspective of diversified investors (accounting beta) or undiversified ones (earnings variability).

Relative Risk Proposition 3: The margin of safety is not a competitor to any of the risk measures above, since it is a post-value adjustment for risk.

Rather than repeat what I said in a much longer post that I had on the topic, let me summarize the points that I made there. When value investors talk about protecting themselves from risk by using a margin of safety, they are talking about building a buffer between value and price, but to use the margin of safety, you need to value a stock first. To get that value, you need a risk measure, and that brings us back full circle to how you adjust for risk, when valuing companies.

Relative Risk in 2020

With that long lead-in, let's take a look at how companies measured up on relative risk measures, at the start of 2020. In keeping with my argument in the last section that you can use alternative risk measures, I will report on three alternative risk measures:

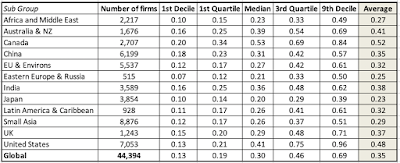
• Betas: I start with betas, estimated with conventional regressions of returns on the stock against a market index, for each of the companies in my sample. To get a measure of how these betas vary across companies, I have a distribution of betas, broken down globally and for regions of the world:
  
  It is worth noting that, at least for public companies, half of all companies have betas between 0.85 and 1.45, globally. If you are wondering why the betas are not higher for companies in riskier parts of the word, it is worth emphasizing that betas are scaled around one, no matter of the world you are in, and are not designed to convey country risk. (The equity risk premiums that I wrote about in my last post carry that weight.)
• Relative Standard Deviation: For those who do not buy into the notion that the marginal investors are diversified and that the only risk that matters is market risk, I report on the standard deviation in stock prices (using the last two years of data):
  
  Note that you can convert these numbers into relative measures, resembling betas, by dividing by the average standard deviation of all stocks. Thus, if you have a US stock with an annualized standard deviation of 35.00% in stock returns, you would divide that number by the average for US equities of 42.36% to arrive a relative standard deviation of 0.826 (=35.00%/42.36%).
• High-Low Risk: For those who prefer a non-parametric and more intuitive measure of risk, I compute a risk measure by looking at the difference between high and low prices in the most recent year, and dividing by the sum of the two numbers. Thus, for a stock that has a high price of 20 and a low price of 12, during the course of a year, this measure would yield 0.25 ((20-12)/ (20+12)). Note that the bigger the range in prices, the more risky a stock looks on this measure, and this too is broken down globally and by region:
  
  As with the other risk measures, this too can be converted into a relative risk measure, by dividing by the average.
• Earnings Variability: Finally, for those who trust accountants more than markets (even though I am not one of them), I have computed a risk measure that is built around earnings variability, computed by looking at the standard deviation in net income over the last 10 years for each firm, and converted into a standardized measure, by dividing by the average net income over the ten years (a coefficient of variation in net income), The global and regional breakdown is below:
  
  The earnings variability number has a bigger selection bias than the other measures, because it requires a longer history (10 years of data) and positive earnings, cutting the sample size down significantly. Here again, dividing a company's coefficient of variation in net income by the average value across all companies will give you a relative risk measure.

I follow up by looking at median values for each of the risk measures by industry grouping. Since I have 94 industry groupings, I will not report them all here, but you can download the data on all of the industry groupings, by clicking here.

Hurdle Rates in 2020

The relative risk measures are a means to an end, since the only reason for computing them is to use them to get to required returns. In this section, I begin by looking at the cost of equity, then bring in the cost of debt and close of by looking at the cost of capital.

a. Cost of Equity

There are three ingredients that go into the cost of equity and the last few posts have laid the foundations for the three inputs:

• The risk free rate is a function of the currency you choose to compute your hurdle rates in, and will be higher for high-inflation currencies than low-inflations ones. Since I will be comparing and aggregating costs of equity across more than 40,000 firms spread across the world, I will compute their costs of equity in US dollars, using the US T.Bond rate as of January 1, 2020, as the risk free rate. You can convert these into any other currency, using the differential inflation approach that I described in my earlier post from a couple of weeks ago.
• The equity risk premium for a company is a function of where it does business, and in my last data update post, I described my approach to estimating equity risk premiums for individual countries, and the process of weighting these (using either revenues or production) to get equity risk premiums for companies.
• For the relative risk measure, I will use betas but as I argued in the last section, I am agnostic about what you prefer to use instead. Thus, if you prefer earnings variabliity as a measure, you can use relative earnings variability as your risk measure.

With these inputs, I estimate the costs of equity for all of the companies in my database, and report the distribution in the table below:

Comparing this distribution to the one for betas, earlier in this post, you will notice a wider spread in the numbers across regions, as we bring in equity risk premium differences into the calculation.

b. Cost of Debt

The cost of debt is a simpler exercise, since it is a measure of the rate at which companies can borrow money today, not a reflection of the rates at which they have borrowed in the past. It is a function of the risk free rate and the default spread:

As with the cost of equity, the risk free rate is a function of the currency in which you estimate the cost of debt in, and I will estimate the costs of debt for all companies in US dollars, again to make comparisons across companies. For the default spread, I have little choice but to use bludgeon measures, since I cannot assess credit risk for 40,000 plus companies. For companies that have an S&P bond rating (about 15% of the sample), I use the rating to estimate a default spread. For the rest, I estimate synthetic bond ratings based on financial ratios (interest coverage and debt ratios). The US $ pre-tax cost of debt distribution is below:

Since these costs are all in US dollars, the differences across regions reflect difference in country default risk and reflect wide divergences. It is worth noting that the tax law tilt towards debt, represented in the fact that interest expenses are tax deductible and cash flows to equity (dividends and buybacks) have to come from after-tax cash flows, is not just a phenomenon for the US, but true over much of the world, with the Middle East representing the holdout. This tax benefit shows up in the cost of capital, through the conversion of the pre-tax cost of debt into an after-tax cost, using the marginal tax rate to make the adjustment:

After-tax cost of debt = Pre-tax cost of debt (1 - Marginal Tax Rate)

In my sample, I use the marginal tax rate of the country in which a company is incorporated. You can find these marginal tax rates, which KPMG should be credited for collecting, also on my website for download.

c. Debt Ratios and Costs of Capital

The final piece of the puzzle in computing the cost of capital is the mix of debt and equity that companies use in funding their operations. In keeping with the cost of capital being a measure of what companies have to pay for their debt and equity today, I use the market values of equity and debt, with leases converted into debt and included in the latter, to compute the cost of capital. While I will talk in more detail about debt loads and choices in a future post, you can sense of the debt load at companies, as a percent of capital (in market value terms) in the table below below:

With these debt ratios, and using the costs of equity and debt also shown above, I compute costs of capital, in US dollar terms, for all publicly traded companies and the resulting distribution is below:

This is a table that I will use, and have already put to use, in valuing companies since it provides a quick and effective way to estimate discount rates for companies, without losing yourself in the details. Thus, when valuing a young, money-losing public company in the US (like Casper, the only mattress-maker that went public last week), I will use a cost of capital of 9.15%, representing the 90th percentile of US firms, whereas to value a slow-growing European company in a stable business,  like Heineken, my cost of capital will be 6.02%, the 25th percentile of European companies. For all companies, the median cost of capital of 7.58% is a good proxy for the number that all companies will converge towards, as they approach maturity. If all of these numbers look low to you, that is because they reflect a risk free rate, in US dollars, that is low, and if it does rise, it will carry these numbers upwards.  As with the risk measures, I have estimated costs of equity, debt and capital, by industry group and you can download them for all companies globally, as well as regionally (US, Emerging Markets, Europe, Japan and Australia/Canada) and for India and China, separately.

YouTube Video


Downloadable Data

1. Industry Average Risk Measures at start of 2020
2. Betas, by industry (Global, US, Emerging Markets, Europe, Japan,  Australia/Canada, India, China)
3. Costs of Debt, Equity and Capital, by industry (Global, US, Emerging Markets, Europe, Japan,  Australia/Canada, India, China)
4. Marginal tax rates, by country, for 2020